- March 8, 2021, 2:00 pm US/Central
- Arka Banerjee, Fermilab
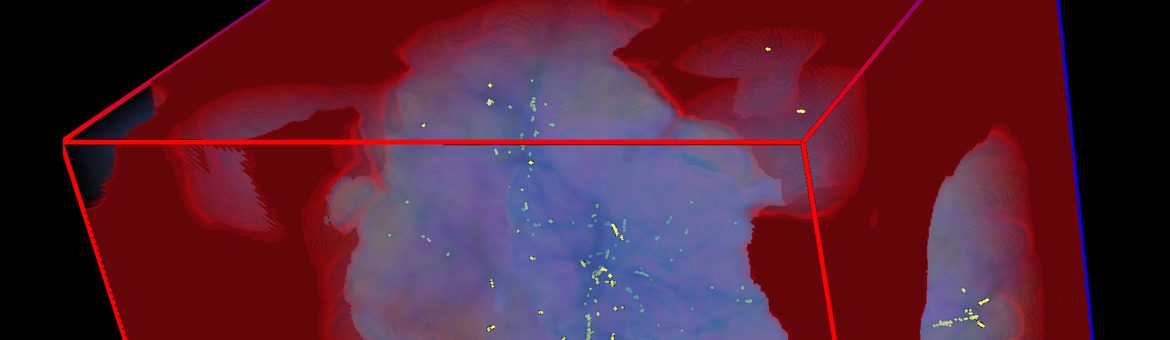
Abstract: The two-point correlation function, or the power spectrum, is the most widely used statistical tool to summarize clustering in the data for cosmological analyses. While the two-point function is a complete statistical description for a Gaussian random field, it does not capture all the information for strongly nonlinear fields relevant for cosmology at low redshifts. In this talk, I will introduce a new set of summary statistics that can be applied to measurements of clustering: the k-Nearest Neighbour Cumulative Distribution Functions (kNN-CDF). I will discuss how to compute this efficiently on discrete datasets, and how these measurements are sensitive to various combinations of all N-point functions in the data. I will demonstrate various applications of these statistics, including the extent to which they are more sensitive to the underlying cosmological parameters, and can produce significantly stronger parameter constraints from the same input data. Finally, I will discuss how nearest neighbor measurements can be applied to joint distributions and cross-correlations of two datasets, and that here too, has greater statistical power than the traditional two-point cross-correlations.
For more information, please contact Yu-Dai Tsai at ytsaiATfnal.gov.